复旦大学《大学物理》课件（英文）-第9章Rotational dynamics(1).pdf

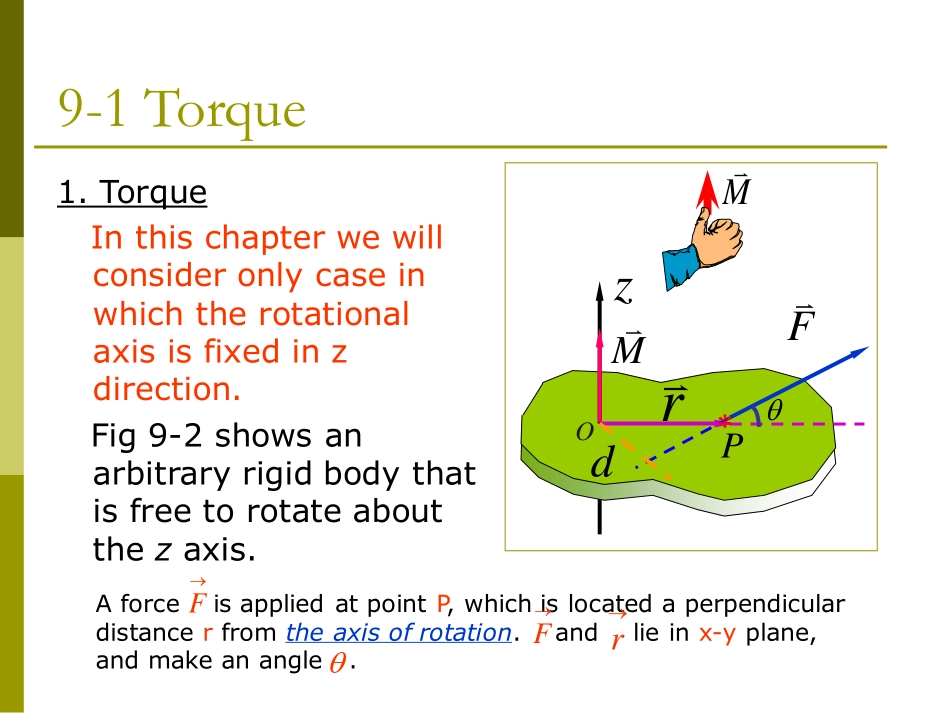
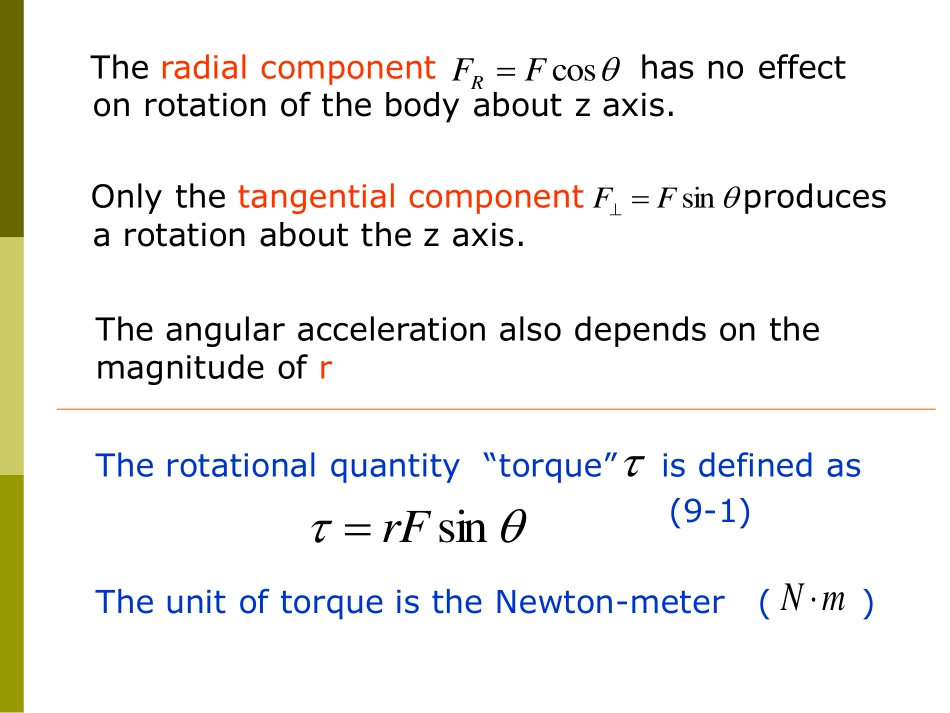
Chapter9Rotationaldynamics9-1Torque1.TorqueInthischapterwewillconsideronlycaseinwhichtherotationalaxisisfixedinzdirection.Fig9-2showsanarbitraryrigidbodythatisfreetorotateaboutthezaxis.PzOMFrdM*AforceisappliedatpointP,whichislocatedaperpendiculardistancerfromtheaxisofrotation.andlieinx-yplane,andmakeanangle.→F→F→rTheradialcomponenthasnoeffectonrotationofthebodyaboutzaxis.Onlythetangentialcomponentproducesarotationaboutthezaxis.cosFFR=sinFF=⊥TheangularaccelerationalsodependsonthemagnitudeofrTherotationalquantity“torque”isdefinedas(9-1)TheunitoftorqueistheNewton-meter()sinrF=mNIfr=0-thatistheforceisappliedatorthroughtheaxisofro...